Versatile Shapes: The Archimedean Semi-Regular Polyhedra

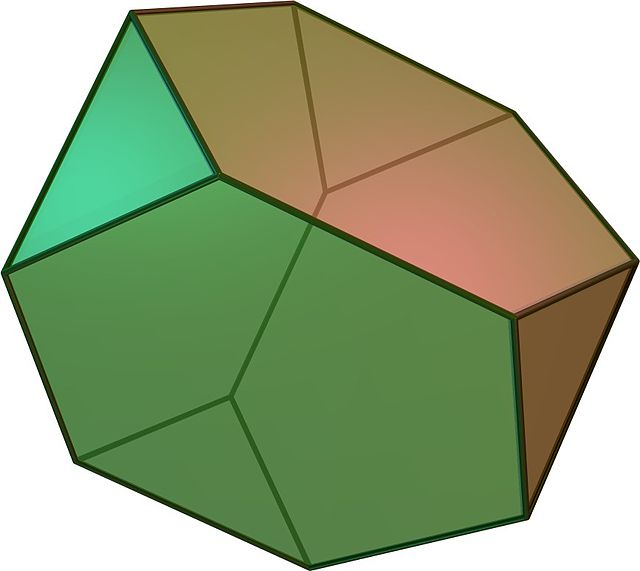
Archimedean semi-regular polyhedra (ASRP) are convex, multi-faceted 3-D objects whose faces are all regular polygons that have sides of equal length and angles of equal degrees. Around every vertex, the same polygons appear in the same sequence, e.g., hexagon-hexagon-triangle in the truncated tetrahedron shown below. Archimedes' original writings that described the 13 ASRP are lost and known only from secondary sources. During the Renaissance, all but one of these polyhedra were gradually rediscovered by various artists. And eventually, in 1619, German mathematician and astronomer Johannes Kepler presented the entire set in his book Harmonices Mundi (The Harmonies of the World).

The Classification of an Archimedean Polyhedron

A key characteristic of the Archimedean solids is that two or more different polygons appear in each of them, unlike the Platonic solids which each contain only a single type of polygon. The polyhedron is required to be convex.

These solids can be fully specified by a vertex configuration, a listing of the faces by number of sides, in order as they occur around a vertex. For example: 3,5,3,5 means that a triangle, pentagon, triangle and pentagon appear in that order around each vertex.

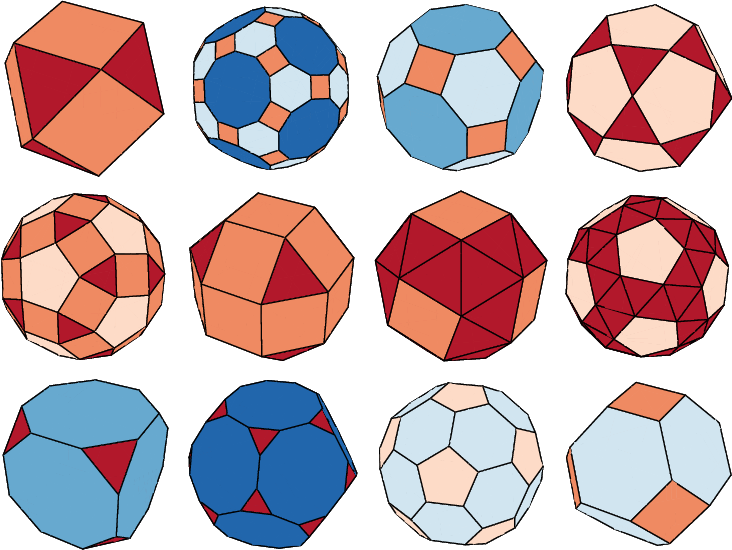
Using this notation, we have the following ASRPs:

• (3, 4, 3, 4) cuboctahedron
• (3, 5, 3, 5) icosidodecahedron
• (3, 6, 6) truncated tetrahedron
• (4, 6, 6) truncated octahedron
• (3, 8, 8) truncated cube
• (5, 6, 6) truncated icosahedron
• (3, 10, 10) truncated dodecahedron
• (3, 4, 4, 4) rhombicuboctahedron, sometimes called the small rhombicuboctahedron
• (4, 6, 8) truncated cuboctahedron, sometimes called the great rhombicuboctahedron
• (3, 4, 5, 4) rhombicosidodecahedron, sometimes called the small rhombicosidodecahedron
• (4, 6, 10) truncated icosidodecahedron, sometimes called the great rhombicosidodecahedron
• (3, 3, 3, 3, 4) snub cube, better called the snub cuboctahedron
• (3, 3, 3, 3, 5) snub dodecahedron, better called the snub icosidodecahedron

The 32-faced truncated icosidodecahedron is particularly interesting. The shape of a soccer ball is based on this solid. Furthermore, the same configuration of shapes was also used for arranging lenses that focused the explosive shock waves of the detonators in the "Fat Man" atomic bomb detonated over Nagasaki, Japan, in World War II.

While the polyhedron names are certainly a pain to write out, there is a certain logic to naming them, adapted from Kepler's Latin terminology. The term snub refers to a process of surrounding each polygon with a border of triangles as a way of deriving for example the snub cube from the cube. The term truncated refers to the process of cutting off corners. To preserve symmetry, the cut is in a plane perpendicular to the line joining a corner to the centre of the polyhedron and is the same for all corners. The process of truncation adds a new face for each previously existing vertex and replaces n-sided polygons with 2n-sided ones. (For example, by comparing the cube and the truncated cube, square faces are replaced with octagonal faces as a result of truncation.) Depending on how much is truncated, different Platonic and Archimedean (and other) solids can be created. If the truncation is exactly deep enough such that each pair of faces from adjacent vertices shares exactly one point, it is known as a rectification.

With this in mind, a more accurate definition of an Archimedean solid would be that it is a convex polyhedron composed of regular polygons such that every vertex is equivalent. Equivalent in this context, means that one can choose any two vertices, for example, x and y, and there is some way to rotate or reflect the entire polyhedron so that it appears unchanged as a whole, yet vertex x moved to the position of vertex y.

Euler's Formula: Finding the Relationship Between Edges and Vertices

Also interesting is that we can use Euler's Formula to figure out the numbers of vertices, edges and polygons from the combination of faces meeting at each vertex. Let S = (p₁, p₂, . . . , p_q) be the given sequence, and m_p be the number of times the number p appears in the sequence. Every vertex is incident with m_p p-gons, and every p-gon has vertices. Then m_pV counts every p-gon times, and the total number of p-gons is given by 

F_p =  m_pV/p

Every vertex is incident with q edges, and every edge is incident with 2 vertices. So qV counts every edge twice, and

E = qV/2

Now,

2 = V - E + F

= V - qV/2 + ∑_p F_p

= V - qV/2 + m_pV/p

Therefore,

2 = V (1 - q/2 + ∑_p m_p/p ),

Once we solve for V, we can use the earlier equations to solve for E and F_p . The total number of faces will be the sum of the F_psquare faces

Prisms and Antiprisms

The Archimedean solids belong to the class of convex uniform polyhedra, the convex polyhedra with regular faces and symmetric vertices, which is divided into the Archimedean solids, the five Platonic solids (each with only one type of polygon face), and the two infinite families of prisms and antiprisms.

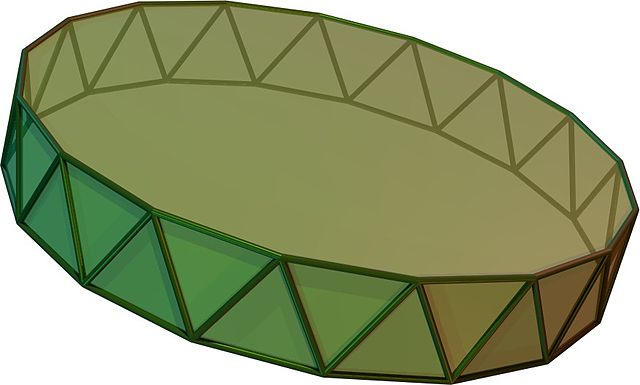
A regular prism is formed by connecting two regular N-sided polygons with N squares, while a regular antiprism is formed by connecting two regular N-sided polygons with 2N equilateral triangles. The Square Prism and the Triangular Antiprism correspond to the Cube and the Octahedron, respectively. The other regular prisms and antiprisms share similarities with Archimedean solids, as they are convex polyhedra with faces composed of regular polygons of two or more types, arranged in the same pattern around each vertex. However, unlike Archimedean solids, regular prisms and antiprisms lack polyhedral group rotational symmetries, such as tetrahedral, octahedral, or icosahedral symmetries.

The Everyday Functions of Polyhedrons

Not only are they interesting to look at, but polyhedrons have many important functions in everyday life. In mathematics, polyhedrons have received a great deal of attention and are used in various fields such as physics, computer graphics, crystallography, architecture, and other disciplines.

Technology and Sound

Take technology as an example, tetrahedrons are frequently applied in electronics, icosahedrons have proven useful in geophysical modelling, and speakers with polyhedral faces radiate sound energy in all directions.

Board Games

To add on, special dice known as polyhedral dice are used in board games, role-playing games, and mathematics games. They are generally applied to add an element of chance or randomness.

Polyhedral dice, in contrast to traditional six-sided dice (D6), have more than six faces, enabling a greater range of outcomes.

Some Examples of Polyhedral dice are:

• D4: A tetrahedron-shaped die with four triangular faces.
• D6: The six-sided die most people are familiar with
• D8: This die has eight triangular faces.
• D20: The twenty-sided die that has twenty equilateral triangular faces.

Chemistry

Polyhedrons have also been a topic of interest in chemistry, where chemists are trying to build molecular polyhedrons, a ground-breaking assembly that has the potential to impact a range of industrial and consumer products, including magnetic and optical materials. Chemists at NYU and the University of Milan have managed to coerce molecules to form a quasi-truncated octahedron, one of the 13 Archimedean solids. As a polyhedron, the structure has the potential to serve as a cage-like framework to trap other molecular species, serving as building blocks for new and enhanced materials. The structure is also remarkably stable given that the hydrogen bonds used to create it are stabilized by the ionic nature of the molecules. In fact, the truncated octahedra assemble further into crystals that have nanoscale pores, resembling a class of well-known compounds called zeolites, which are made from inorganic components.

Conclusions

The beauty of mathematics is everywhere around us. In the case of the Archimedean solids, not only are they fascinating to look at, but they also wield the power to shape our world (pun intended). The possibilities are endless when mathematics is combined with other fields of research and innovation. From architecture to art, and from physics to engineering, the principles underlying Archimedean solids have influenced designs and structures that are both functional and aesthetically pleasing. Their symmetrical beauty offers insights into natural forms, like crystals and viruses, while also facilitating the creation of complex networks, optimization algorithms, and even cutting-edge materials.

Ultimately, the fusion of mathematics with other disciplines fosters innovation that pushes the boundaries of what we thought was possible. The Archimedean solids are a reminder that the world of mathematics is not limited to the pages of textbooks but is an active, driving force that shapes our understanding of the universe and the progress of humanity. The endless possibilities they represent are a testament to the power of mathematical thinking in unlocking new knowledge and creativity.