The Quadratic Formula: What Is It and Where Did It Come From?

It is safe to assume that many of us are familiar with the quadratic formula, which allows one to find the root(s) of quadratic equations in the form of ax²+bx+c=0. A quadratic equation is a polynomial equation in which the highest power of x is exactly 2. In other words, it is a polynomial equation of degree 2.

More formally, a quadratic equation is written as follows: y=ax²+bx+c. (a≠0, b and c are both real numbers.) In which different values for x produce different values for y. This can be represented by graphing the function. In mathematics, a function is something that takes an input and produces only one possible output for that given input. Therefore, y=ax²+bx+c is an example of a function as it relates an input (x) to a singular output (y).

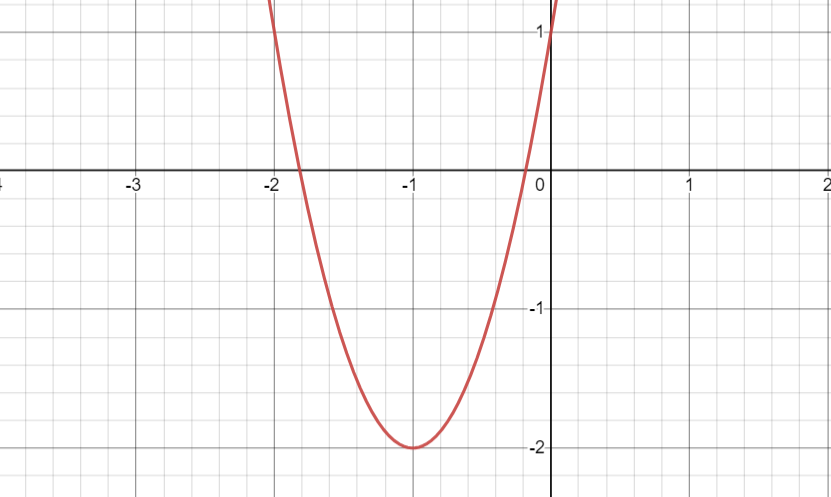
The graph of a quadratic function is a curve called a parabola. The x-intercept is also an important concept in mathematics and graphing because it represents the point where a function or equation crosses the x-axis. The x-intercept(s) is also referred to as the root(s) of a quadratic equation. The roots of a quadratic equation are the two values of x, which are obtained by solving for 0. To do this, however, the quadratic equation must be written in standard form: ax²+bx+c=0. (The x-intercept(s) is the point at which the y-value is 0.)

The quadratic formula is a very useful tool for finding the roots of a quadratic equation. It is a formula that can solve quadratics that do not factor easily or at all:

The x-intercept(s) of a function differs from time to time. This is determined by the value under the square root in the quadratic formula, b²-4ac, which is called the discriminant. Suppose the discriminant is positive, the quadratic will have two real solutions or two x-intercepts. If the discriminant is zero, then the quadratic only has one real solution or one x-intercept. If the discriminant is negative, then the quadratic has no real solutions. This explanation makes sense because the square root of a negative number does not exist. Therefore, there is no x-intercept.

Δ = discriminant = b²-4ac

Δ > 0 ⇒ 2 solutions 

Δ < 0 ⇒ no solutions

Δ = 0 ⇒ 1 solution

The quadratic formula is actually derived using the steps involved in completing the square:

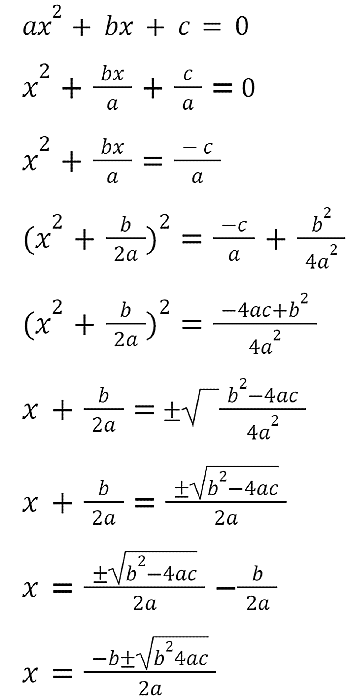
Step 1: To remove the coefficient of x², divide both sides of the equation by a.

Step 2: Subtract c/a from both sides of the equation.

Step 3: Express the left side of the equation as the square of a binomial whilst adding b²/4a² to the other side of the equation. This is so that both sides of the equation remain equivalent.

Step 4: Turn the -c/a and b²/4a² into equivalent fractions and add them together.

Step 5: Find the square root of both sides of the equation.

Step 6: Simplify the right side of the equation.

Step 7: Subtract b/2a from both sides of the equation.

Step 8: Simplify the right side of the equation.

While seemingly simple, the quadratic formula has been derived in the course of a few millennia, and like most historical developments of mathematics, it was not a linear process.

Many mathematical developments come about as solutions to a problem, whether it be theoretical or practical. In the case of the quadratic formula, the original problem arose around 2000 BC. During this time, Egyptian, Chinese, and Babylonian engineers were of great use to society as they knew how the area of a square scales with the length of its side. Furthermore, they knew how to calculate the area of more complex designs such as rectangles and T-shapes. The skill they lacked was calculating the length of the sides, starting from a given area which was something their clients often needed.

In around 1500 BC, Egyptian wisemen came up with a way to overcome this problem using a quick yet impractical solution. Instead of learning an operation or a formula to calculate the side lengths of a shape from its area, they calculated the area for all possible sides and shapes of squares and rectangles. It is important to note that Egyptian mathematics did not contain the equations and numbers that we know today, therefore, coming up with a "simple formula" was almost unheard of. Egyptian engineers would use this table as a reference for certain builds, such as a loft to store a certain capacity of papyrus. This method worked fine until a more general solution was needed. At this point, Babylonian mathematics had an advantage over Egyptian mathematics because they used a number system, much like the one we use today. As a result, they found a more general method to solve problems involving areas, namely the "completing the square" method.

Thanks to mathematical geniuses such as Pythagoras and Euclid, a strictly geometric attempt was made to find a more general formula for solving quadratic equations. Pythagoras concluded that the ratios between the area of a square and its respective side length were not always whole numbers, however, refused to accept proportions other than rational. Euclid, on the other hand, went as far as to say that this proportion might also not be rational and that irrational numbers existed as well. Even with this information, it was not possible to calculate the square root of any number by hand with a good approximation for the exact value which is what architects and engineers needed.

Hindu mathematics, on the contrary, was substantially advanced in comparison to the system put in place during 300 BC for it had used the decimal system since at least 600 AD. Zero was also an important number for it could make numbers infinitely bigger or infinitely smaller. As a result, a Hindu mathematician called Brahmagupta managed to devise the general solution for the quadratic equation using numbers. He also recognised two roots in the solution, as every positive integer has a negative square root and a positive square root. However, it was another Hindu mathematician called Baskhara who created the final, complete solution as we know it today. An alternative derivation of the quadratic formula came around 820 AD by a famous Islamic mathematician, Musa Al-Khwarismi. His algebra was entirely rhetorical and rejected negative solutions; it was first introduced to Europe by Jewish mathematician Abraham bar Hiyya who lived in Barcelona around 1100 AD.

During the Renaissance in 1500 AD, mathematics made a comeback to the intellectual scene. By 1545, Girolamo Cardano, one of the best algebraists of his time, compiled the works of Al-Khwarismi and Euclid related to the quadratic equations. He allowed for the existence of complex and imaginary numbers - roots of negative numbers - in his works. François Viète, a French mathematician, introduced mathematical notation and symbolism. This giant step for mathematics was fostered by René Descartes who published La Géométrie which many claim contributed to the birth of modern mathematics and the formation of the quadratic formula we know of today.

The quadratic formula is a versatile mathematical tool; Its practical applications span across multiple fields, including physics, engineering, finance, computer graphics, economics, biology, and geometry. It is used to solve real-world problems involving motion, structures, financial modeling, computer-aided design, population dynamics, and geometric relationships. The formula is crucial for determining critical points, making it a valuable tool in problem-solving and analysis across diverse disciplines.