Trigonometry: Its Fascinating Origins and Applications

Trigonometry is the branch of mathematics that deals with the study of the relationship between the sides and angles of a right-angle triangle. Thus, it helps in finding the measure of unknown dimensions of a right-angled triangle using trigonometric formulas, functions, or trigonometric identities. There are six functions of an angle that are commonly used in trigonometry: sine (sin), cosine (cos), tangent (tan), cotangent (cot), secant (sec) and cosecant (csc). Learning these functions and their respective formulas is vital to understanding the concept of trigonometry.
The Basics of Trigonometry
Before learning the trigonometric functions, one must be able to identify the different sides of a right-angle triangle.

A right-angled triangle has the following three sides:
Hypotenuse (r): This is the side opposite the right angle and the triangle's longest side.
Adjacent (x): This is the adjacent side to the angle θ
Opposite: (y): This is the side opposite to the angle θ
(Note that the position of the opposite and adjacent can change depending on where the angle θ is located. The adjacent side is not always the base of the triangle.)
A helpful mnemonic for remembering the definitions of trigonometric functions is given by "SOHCAHTOA", i.e., sine equals opposite over hypotenuse, cosine equals adjacent over hypotenuse, and tangent equals opposite over adjacent.
sin θ = opposite/hypotenuse
cos θ = adjacent/hypotenuse
tan θ = opposite/adjacent
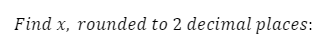
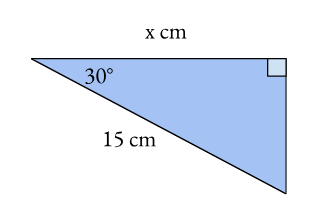

If we know two side lengths of a right-angled triangle, we can use trigonometry to find the angles. For example:

The Trigonometry Table
The trigonometric table is a collection of values of trigonometric functions of various standard angles including 0°, 30°, 45°, 60°, and 90° in the format of a table. This table can be used as a reference to calculate the trigonometric values for various other angles given the patterns existing within trigonometric ratios and angles.

The Unit Circle and Supplementary Angles
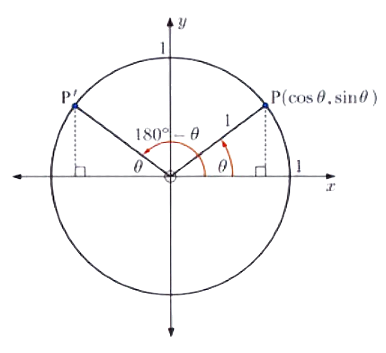
Two angles are supplementary if they add up to 180°. The sine, cosine and tangent of supplementary angles have a certain relation. If α and β are supplementary angles then we have:
sin (α) = sin (β)
cos (α) = - cos (β)
tan (α) = - tan (β)
That is to say that their sines are equal and their cosine and tangent are equal with opposed signs.
To better understand the relationship between the sines and cosines of supplementary angles, we use what is called the unit circle. A unit circle is a circle with a radius measuring 1 unit and is algebraically represented using the second-degree equation with two variables x and y. It is used to find the values of the trigonometric ratios sin, cosine, and tangent.
The equation of a unit circle is x2+y2=1
This is the simplified version of (x - a)2 + (y - b)2 = r2.
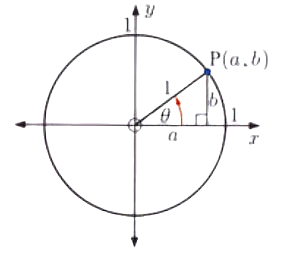
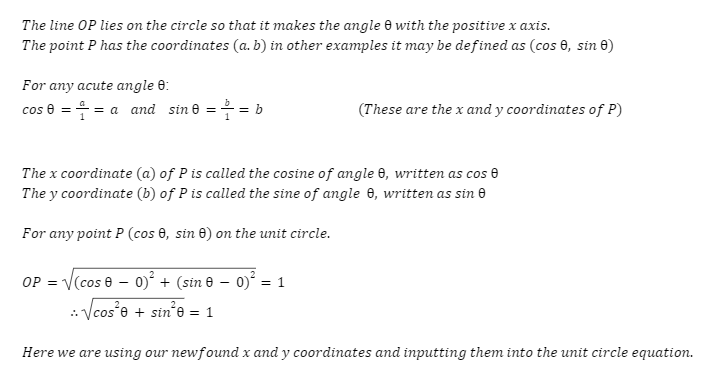

While the examples displayed above are purely theoretical, the unit circle has various applications within the fields of mathematics, science, and engineering. It is used in physics to describe the circular motion of objects such as orbiting planets. In engineering, it is used to design machines and structures that involve rotating components.
Trigonometric Functions
Trigonometric functions have different properties such as domain and range which can be studied using the trigonometric function graphs. Below are the graphs of the basic trigonometric functions sine and cosine.
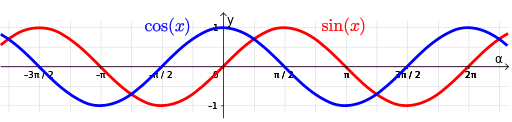
Sin (θ): Domain (-∞,+∞); Range (-1,+1)
Cos (θ): Domain (-∞,+∞); Range (-1,+1)
The trigonometric functions are periodic, with a period of 360 degrees or 2π radians, except for the tangent and the cotangent, which have π as the smallest period. This means their values repeat at those intervals.
A Brief History of Trigonometry
The word trigonometry comes from the Greek words trigonon ("triangle") and metron ("to measure") and until the 16th century, it was primarily concerned with computing the numerical values of the missing dimensions of a triangle when the values of the other parts were given. Calculations like these are what distinguish trigonometry from geometry, which mainly deals with qualitative relations.
Trigonometry can trace its origins back to ancient Egypt, Mesopotamia, and the Indus Valley over 4000 years ago. However, the first recorded use of trigonometry came from the Greek astronomer Hipparchus who is credited with being the founder of trigonometry. It all started in the form of a circle from which he compiled a table of numbers where the lengths of chords of a circle were related to the length of the radius. This table was made to aid his astronomical calculations and proved to be very useful in other fields as well.
Ptomely, another Greek astronomer of the time, made improvements to the table to observe planetary motion and published it in his major work called the Almagest, a thirteen-book compendium on astronomy, which was a tool used by fellow astronomers for the next 1000 years and also found its way to Hindu and Arab scholars. On such scholar was Aryabtha, a Hindu mathematician of the 5th and 6th century AD, who constructed a table of the lengths of half-chords of a circle with a radius of one unit. This became the first table of sine values. Later on, in the tenth century, the Persian mathematician and astronomer Abul Wáfa introduced the tangent functions and improved methods of calculating trigonometry tables.
Trigonometry was further expanded in the 16th century when Georg Joachin de Porris, also known as Rheticus, produced comprehensive tables of the aforementioned six trigonometric ratios with remarkable accuracy. He managed to achieve tedious calculations without the aid of a calculator or a computer. It was also at this point that geometry and trigonometry became two distinctive branches of mathematics.
Of course, delving into the complicated realm of trigonometry would take several articles. Therefore, it is important to note that the different aspects and applications of trigonometry are not limited to the ones listed in this article. Not only are the theoretical implementations fascinating, but so is the story behind how they came about. Trigonometry is still taught in high school classes today because of its versatility and practicality in different fields of study and practice. The principles of trigonometry are essential in solving problems, measuring distances, and understanding the world around us.



