The Mathematical Paradoxes of Greek Philosopher Zeno of Elea


The Origins of Zeno's Paradoxes
The origins of paradoxes and how Zeno of Elea contributed to their development.
The Origins of Paradoxes
The term paradox comes from the Greek para ("contrary to") and doxa ("opinion). The exact definition of the word has evolved over time. Originally, a paradox was something contrary to common opinion, and later became a term used for something that contradicted common sense. In modern terms, a paradox is an apparently true statement or group of statements that ultimately leads to a contradiction.
The etymology of paradox can be traced back to Plato's famous dialogue Parmenides where Zeno of Elea coined the term "paradoxon" to support the claims of said work: " that real existence is indivisible, which means it is immobile, immutable, and permanent; the movement, changes, and multiplicity of the world are illusory perceptions based upon sense experiences; truth is accessible by reason alone." (Zeno of Elea, New World Encyclopedia)
Zeno of Elea (c. 495 BCE - c. 430 BCE)
Zeno of Elea was a Greek philosopher and mathematician and is especially known for his paradoxes and contribution to the development of logical and mathematical reasoning as well as the concepts of continuity and infinity.
He was a student of Parmenides and a member of the Eleatics, a group of pre-Socratic philosophers in the 5th century BC centered around the ancient Greek colony of Elea. Little about his life is known for certain apart from the fact that he was from Elea and a devout believer in monism, the belief that all reality is one single indivisible object; the only primary source that gives us a little insight into his life is the dialogue Parmenides by Plato which takes place when Zeno is about 40 years old. In this work, Zeno is portrayed as a zealous defender of his instructor Parmenides who wished to prove that belief in the physical world is more absurd than belief in the Eleatic idea of a single entity of existence. Plato also has Socrates hint at a previous romantic relationship between Parmenides an Zeno. It is unsure just how accurate the depiction of Zeno is, however, many agree that it bears at least some truth.
Zeno died in c. 430 BC. According to Diogenes Laërtius, who was a biographer of the Greek philosophers, Zeno was killed while engaged in a plot to overthrow the tyrant Nearchus. He was apparently captured and killed after he refused to give the names of his co-conspirators. Before his death, Zeno is rumored to have asked to whisper the names into Nearchus's ear, only to bite the ear when he approached, holding on until he was killed.
Since then, the writings of Zeno have been lost. While there are no existing fragments of his original thoughts, our modern understanding of Zeno's philosophy comes through recording by his subsequent philosophers; one of them being Aristotle who recorded Zeno's Paradoxes in his book Physics.

Zeno's Paradoxes
Zeno of Elea (c. 495 BCE - c. 430 BCE)
Zeno's paradoxes question the concepts of space and time; the relationships between logical reasoning and sense of experience; the meaning of reality; and the concepts of finite and infinite.
Dichotomy Paradox
That which is in locomotion must arrive at the half-way stage before it arrives at the goal. — as recounted by Aristotle, Physics
Say that Atalanta wishes to walk to the end of a path. Before she can get there, she must get halfway there. Before she can get halfway there, she must get a quarter of the way there. Before traveling a quarter, she must travel one-eight, before an eighth, one-sixteenth.
This predicament can be represented using the following sequence:
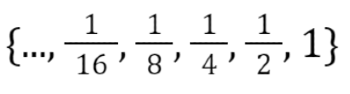
Two problems arise as a result of this sequence. Firstly, it requires one to complete an infinite number of tasks which Zeno argues is an impossibility. Secondly, the sequence contains no first distance to run, as any possible first distance could be divided in half, and therefore would not be first after all. As a result, the trip cannot even begin.
Zeno concludes that travel over any finite distance cannot be completed nor begun, and so all motion must be an illusion.
Achilles and the Tortoise
In a race, the quickest runner can never overtake the slowest, since the pursuer must first reach the point whence the pursued started, so that the slower must always hold a lead. — as recounted by Aristotle, Physics
Say that Achilles is in a footrace with the tortoise. Achilles gives the tortoise a head start of 100 meters. Suppose that each racer starts running at some constant speed, Achilles being the faster one.
After some finite time, Achilles will have run 100 meters, bringing him to the tortoise's starting point. During this time, the tortoise has run a much shorter distance, for instance, 2 meters. It will then take Achilles further time to run that distance, by which time the tortoise will have advanced farther. The pattern continues, whenever Achilles arrives somewhere the tortoise has been, he still has some distance to go before he can reach the tortoise.
Arrow Paradox
If everything when it occupies an equal space is at rest at that instant of time, and if that which is in locomotion is always occupying such a space at any moment, the flying arrow is therefore motionless at that instant of time and at the next instant of time but if both instants of time are taken as the same instant or continuous instant of time then it is in motion. — as recounted by Aristotle, Physics
While the first two paradoxes divide space, this paradox divides time; not into segments, but into points.
An arrow that is stationary in space is practically indistinguishable from one that is flying through space, given that one only looks at a snapshot of it.
According to Zeno, for any motion to occur, an object must change the position that it occupies. He states that at any one instant of time, the arrow is neither moving to where it is nor to where it is not. It cannot move to where it is not, because no time elapses for it to move there; it cannot move to where it is, because it is already there. So at every instant of time, there is no motion occurring. Therefore, if everything is motionless at every instant, and time is composed of instants, then motion is impossible.

Proposed Solutions to the Dichotomy Paradox
How physics can be used to solve Zeno's paradoxes
The oldest recorded solution to the dichotomy paradox was done purely from a mathematical perspective. The claim admitted to there being an infinite number of times Atalanta would have to divide her starting distance by half, making each new distance smaller and smaller than the prior one. However, as long as one could prove that the total sum of each distance adds up to a finite value then it does not matter how many small distances there are.
The aforementioned sequence does in fact converge to 1, so adding an infinite number of terms still allows one to cover the entire needed distance.
However, this reasoning is flawed as it does not disclose how long it would take one to reach the destination. There is no guarantee that each of the infinite distances occurs in a finite amount of time. If each distance took the same amount of time to cover, regardless of how big or small, then it would take an infinite amount of time to cover the total distance.
A solution was proposed by Archimedes who asserted that it must take less time to complete a smaller distance than it does to complete a larger distance, therefore covering the finite distance would take only a finite amount of time. This line of logic was also flawed; sure, the time it takes to finish each step will decrease, but the total journey would still take an infinite amount of time. [½ + ⅓ + ¼ + ⅕ + ⅙ + ...] does not have a limit as it is a diverging series.
Evidently, trying to solve the paradox from purely a mathematical perspective leads to further contradictions.
In essence, though, the paradox is about motion which, by definition, is the amount of distance covered in a specific amount of time. Speed is how fast something moves; velocity is how fast something moves in a stated direction. It is the overall change in distance divided by the overall change in time. There are different types of calculable velocities. There is constant velocity, without acceleration; changing velocity, with acceleration; instantaneous velocity, the velocity at one specific moment in time; and average velocity, the velocity over a certain or whole part of a journey.
The relationship between distance, velocity, and time is stated as follows: distance = velocity × time. This applies to an object in constant motion.
Newton's first law states that objects at rest remain at rest and objects in motion remain in constant motion unless acted upon by an outside force. This is the resolution of the Zeno paradox. If Atalanta is walking at a steady pace, she will cover each half of the distance in less and less time because her speed stays the same. Each time she halves the distance, it takes her only half the time to cover it. So, even though she keeps dividing the distance into smaller parts, she will eventually reach her destination in a finite amount of time because her speed does not change. Therefore, motion is not an illusion as proposed by Zeno.
Conclusion
At the end of the day, paradoxes are not meant to be solved. Rather, they are contradictions that present logical challenges. In fact, the several arguments proposed by philosophers and mathematicians in the last two millennia did not and have not managed to solve Zeno's paradoxes. They have only managed to propose other methods to work out the correct result, such as the mathematical resolution to the paradox of Achilles and the Tortoise.
Regardless, paradoxes are fascinating to think about and discuss with others. They challenge us to question the logical workings of our world and find creative and abstract ways of explaining them.
“A bad feeling is a commotion of the mind repugnant to reason, and against nature.” – Zeno of Elea
Links:



