Napoleon's Theorem: The Amazing Properties of Triangles

The Hidden Side of Napoleon Bonaparte
Upon first hearing the name Napoleon Bonaparte, one might think of the great military commander who seized the position of Emperor of France following the French Revolution in the 18th century. During his reign, he introduced numerous political and societal reforms, including reconstructing the French educational system, supporting a social order based on merit and spreading the French Revolutionary ideas across Europe and ultimately, the world.
However, lesser known to the common folk is that of Napoleon's achievements in the field of mathematics. Numerous accounts report that mathematics was the subject Napoleon excelled at the most during his education, resulting in him building a career in the artillery wing of the French army. In his later years, Napoleon had weekly meetings with the greatest mathematicians of his time including Lagrange, Laplace, and Legendre. In fact, he had such a great understanding of the subject that there exists a famous trigonometric proof bearing his name – Napoleon's Theorem, although many are unsure whether or not he had anything to do with the creation of this theorem.
Napoleon's Theorem Proofs
The theorem goes as follows: Take any triangle, and for each edge construct an equilateral triangle with that edge as its base. The three centroids of the triangles so constructed define an equilateral triangle. The difference in areas of the outer and inner triangles equals the area of the original triangle.
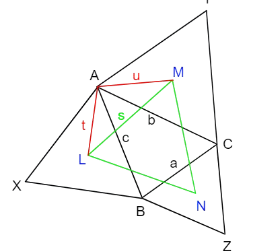
In the figure above, △ABC is the original triangle. △AXB, △BZC, and △CYA are equilateral triangles constructed on their sides' exteriors, and points L, M, and N are the centroids of those triangles. The theorem for outer triangles states that triangle △LMN is equilateral.
But how can we be sure that △LMN is equilateral? Well, there are many proofs of the theorem's statement. However, only two of them will be discussed in this article.
Proof 1: "Hammer and Tongs" Trigonometry
To make this as clear as possible, we use standard notations when labelling the figure above: in the ΔABC, A denotes both the vertex A and the corresponding ∠A, a is both BC and its length. In addition, let L denote the centroid of the equilateral triangle on side AB, and M denote the centroid of the equilateral triangle on side AC, etc. Let s denote the length of segment LM, t the length of segment AL, and u the length of segment AM.
The centroids L and M are separated by an angle of 30∘ from their respective sides. This comes from the internal angles in the equilateral triangles, which are each 60∘.
We apply the Law of Cosines to calculate the length s2 between centroids L and M:
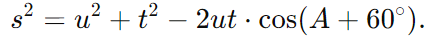
The centroid of an equilateral triangle lies along the median, at a distance 2/3 from the vertex to the midpoint of the opposite side.
Using the height of an equilateral triangle, the distances from vertex A to centroids L and M are:
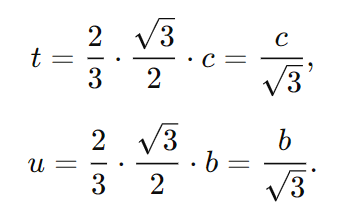
Substituting the expressions for t and u into the Law of Cosines equation, we get:
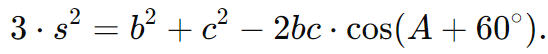
Expand cos(A+60∘):
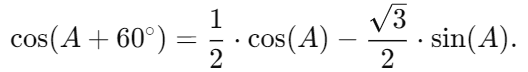
Substituting this into the equation, we get:
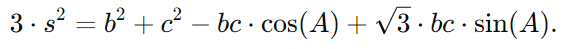
Also, from the formula for the area of triangle ΔABC:

Substituting the Law of Cosines and the area expression into the equation for s2, we get:
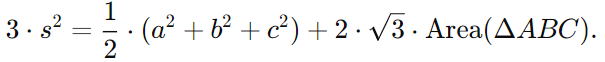
Since this equation is symmetrical in a, b, and c, it follows that the triangle formed by the centroids L, M, and the centroid of the equilateral triangle on side BC is equilateral.
Proof #2: Proof with Complex Numbers
Given a triangle ΔABC, we construct three equilateral triangles on its sides:
- Equilateral triangle ΔABC1 on side BC.
- Equilateral triangle ΔBCA1 on side CA.
- Equilateral triangle ΔCAB1 on side AB.
We will use complex numbers to represent the vertices of these triangles. Let the complex numbers corresponding to points A, B and C be denoted by A, B, and C respectively. Similarly, the points C1, A1 and B1 are the third vertices of the equilateral triangles built on sides BC, CA, and AB, respectively.
To capture the symmetry of equilateral triangles, let j represent the complex number corresponding to a rotation by 120°. This means:
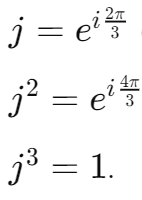
We can use the property that rotating a point by 120° counterclockwise multiplies its complex coordinate by j. Therefore if we know two vertices of an equilateral triangle, we can express the third vertex using this rotation.
The fact that ΔABC1, ΔBCA1 and ΔCAB1 are equilateral can be expressed using rotations as follows:
- For equilateral triangle ΔABC1 on side BC, the vertices satisfy:
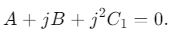
- For equilateral triangle ΔBCA1 on side CA, the vertices satisfy:
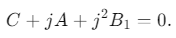
- For equilateral triangle ΔCAB1 on side AB, the vertices satisfy:
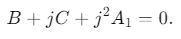
The centroids of the three equilateral triangles are:
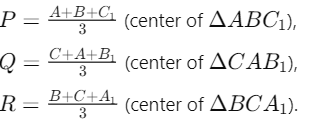
Goal:
We want to show that these centroids satisfy the following equation:
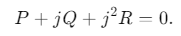
Proof:
We begin by multiplying the equation by 3 to eliminate the denominators:
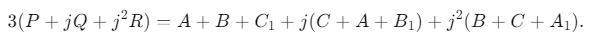
Substitute the expressions from the equations of the equilateral triangles:
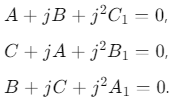
When we substitute these into the original equation we get:
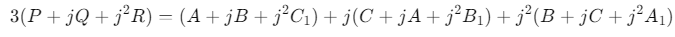
Since each of the terms in the brackets equals 0 by the conditions for the equilateral triangles, we have:

which gets us to:
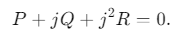
Thus, the sum of the centroids of the three equilateral triangles, weighted by powers of the rotation j, equals zero. This proves that the centroids of the equilateral triangles form equilateral triangles themselves, which is Napoleon's Theorem.



