The Enigma of Infinity: Hilbert's Paradox

I have always enjoyed researching and learning about mathematical paradoxes. Partly because they are interesting to think about, and partly because they make us question our already-existing knowledge about the world around us. The Infinite Hotel Paradox or Hilbert's Paradox of the Grand Hotel is one such paradox, illustrating a counterintuitive property of infinite sets. It was introduced by German mathematician, David Hilbert, in his 1925 lecture "Über das Unendliche" and came to popular attention through George Gamow's book "One Two Three... Infinity" (1947).

What Does an Infinite Hotel Look Like?

Imagine a hotel where there are infinitely many rooms with infinitely many guests. (The manager, maids and construction workers are working around the clock. The only silver lining is their monthly income, which amounts to infinite dollars, even after taxes.) The rooms are numbered 1, 2, 3, and so on with no upper limit, making it a countably infinite number of rooms. Initially, every room is occupied, and yet, new visitors arrive, each expecting their own room. Now usually, a finite hotel would not be able to accommodate new guests once every room is full. However, Hilbert showed that in the infinite hotel, even an infinite number of existing guests and newcomers can each have their own room.

Finitely Many New Guests

Suppose one additional guest wanted to stay at Hilbert's hotel (the Infinite Hotel). Hilbert suggested that the hotel can accommodate them and the existing guests if infinitely many guests simultaneously move rooms. This is to say that the guest currently in room 1 moves to room 2, the guest currently in room 2 moves to room 3, and so on. In other words, every guest moves from their current room n to room n + 1. As Hilbert's hotel has no final room, every guest has a room to go to. After all of the guests have been moved, room 1 is empty and the new guest can be moved into that room. So, by repeating this process, it is possible to make room for any finite number of new guests. If one were to express this using variables, one could say that when k (the number of finite new guests) seeks a room, the hotel can apply the same procedure and move every existing guest from room n to room n + k.

But what about if infinitely many new guests wanted a room at Hilbert's hotel?

Infinitely Many New Guests

Hilbert argued that it is also possible to accommodate a countably infinite number of new guests. He could free up an infinite number of rooms by asking the guests to move to the room number which is double their current one, leaving the infinitely many odd-numbered rooms free. The person occupying room 1 would move to room 2, the guest occupying 3 would move to 6 and so on. So, in general, the guest occupying room n would move to room 2n (2 times n). Of course, practically speaking, the guest occupying room 1 276 456 would not be too happy.

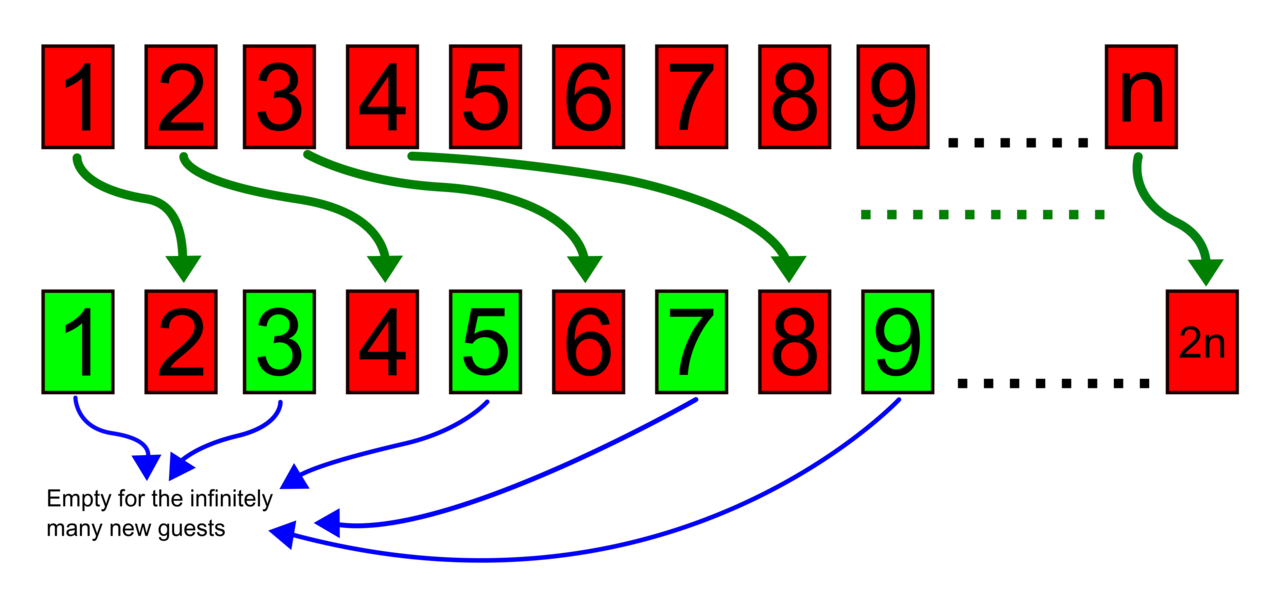
Infinitely Many Buses with Infinitely Many Guests Each

Now what if, say, an infinite line of infinitely large buses, each with a countable infinite number of passengers, pulls up to the hotel to rent rooms? Hilbert, with the help of Euclid's theorem (a statement in number theory that asserts that there are infinitely many prime numbers), devised a way to accommodate the new guests. To accomplish this task, he suggested that each current guest of the hotel be assigned to the first prime number, 2, raised to the power of their current room number. So, the current occupant of room number 8, would go to room number 2⁸ which is room 256. So, in general, the guest occupying room n would move to room 2ⁿ

Hilbert then takes the passengers on the first of the infinite buses and assigns them to the next prime number, 3, raised to the power of their seat number on the bus. (This is possible because there are a countably infinite number of passengers on each bus.) So, the person in seat number 8 on the first bus would go to room number 3⁸ which is room 6561. In other words, the guest occupying room n would move to room 3ⁿ. This process is repeated for all of the passengers on the first bus.

The passengers on the second bus are assigned powers of the next prime, 5. The following bus is assigned powers of 7, then the next bus is assigned powers of 11, and so on. This is possible because each of these numbers only has 1 prime number base raised to a natural number, meaning that there are no overlapping room numbers. The only problem, here, is that many rooms will go unfilled. For instance, room number 6, since 6 is not a power of any prime number.

The Countable Infinity of Natural Numbers vs Real Numbers

Hilbert's strategies are only possible because the Infinite Hotel deals with the lowest level of infinity, namely, the countable infinity of natural numbers. In the case of Hilbert's Hotel, we use natural numbers for the room numbers and seat numbers on the buses.

If we were to deal with the infinity of real numbers, the hotel would have no beginning, end, or even middle, as there would be no way to include every number systematically. Real numbers are the set of both rational and irrational numbers; so all natural numbers, negative numbers, decimals, and fractions come under this category. With this in mind, there would be an infinite number of rooms in the basement to include -3, -√4, and -267. There would even be fractional rooms, such as 1/2, 3/1846 and 4/92. And there would even be rooms like √2 and π.

So what are the different kinds of infinity?

The Different Kinds of Infinity

At the end of the 19th century, Russian-born mathematician, Georg Cantor, pioneered the study of infinite sets and infinite numbers, asserting that they can be of various sizes.

The aforementioned infinity of natural numbers, though infinitely numerous, is actually less numerous than the infinity of real numbers. Cantor showed that there are more real numbers between zero and one than there are numbers in the entire range of naturals. He did this by way of contradiction. He assumed that the two infinite sets were the same size, then used logical reasoning to find a flaw that goes against that assumption.

He reasoned that the naturals and the 0-1 subset of the reals have equally many members, implying that the two sets can be put into one-to-one correspondence.

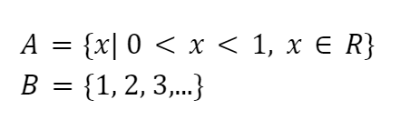
In this case, a one-to-one correspondence between sets A and B is a similarly pairing of each object in A with one and only one object in B. If the elements of two sets can be paired so that each element is paired with exactly one element from the other set, then there is a one-to-one correspondence between the two sets. The two sets are said to be equivalent.

Cantor used this idea to compare natural numbers (like 1, 2, 3, etc.) with real numbers between 0 and 1 (like 0.5, 0.75, etc.). He imagined pairing each natural number with a unique real number. This would mean you could list the real numbers in a sequence: r₁, r₂, r₃, and so on, where r_n is the real number paired with the natural number n.

Then, Cantor came up with a clever trick. He created a new real number, called p, by ensuring that each digit of p is different from the corresponding digit in each rn. For example:

• If the first digit of r₁ is 4, p's first digit is not 4 (say 3).
• If the second digit of r₂ is 7, p's second digit is not 7 (say 4).
• If the third digit of r₃ is 1, p's third digit is not 1 (say 4).

Continuing this way, Cantor constructed p so that it differs from every listed real number in at least one decimal place. Because p is different from all the listed real numbers, it shows that not all real numbers between 0 and 1 can be paired with natural numbers.

This proves that there are more real numbers between 0 and 1 than there are natural numbers. Real numbers are "uncountably" numerous, meaning their infinity is larger than the infinity of natural numbers. This was a groundbreaking idea because it showed different sizes of infinity, something not considered before.

The lowest level of infinity is that of the countable infinity of natural numbers. However, it may come as a surprise that many other infinite sets are actually countable. For instance, the set of integers, which are composed of the natural numbers, their negative counterparts, and zero. While many assume this set to be bigger than the naturals, there is a way to assign exactly one integer to each natural number (proving one-to-one correspondence) by bouncing back and forth between positive and negative numbers. Doing this proves that the two sets are the same size, making them both countably infinite.

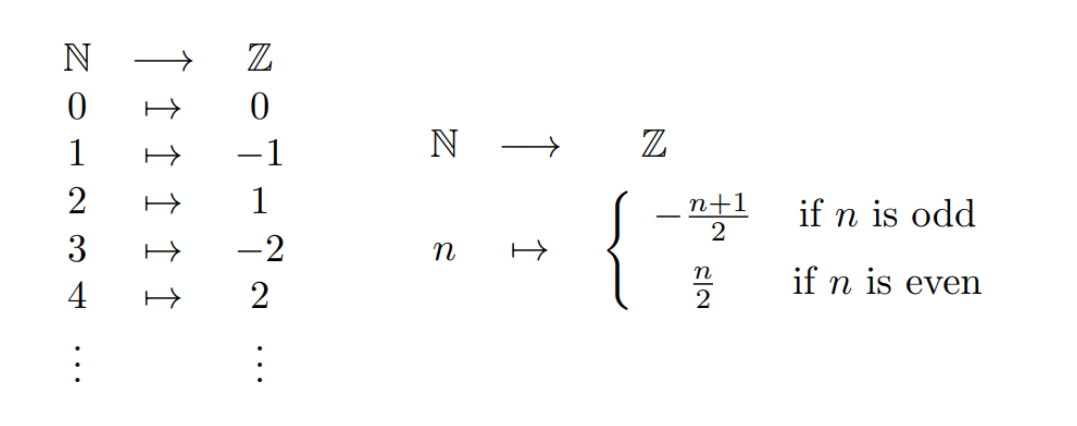
Cantor called the countable infinite, the infinity of all counting numbers,  ℵ_0.  ℵ is the Hebrew letter aleph. The number of points on a line segment, a bigger infinity, is denoted by ℵ_1.  Yet, there is an even bigger infinity, ℵ₂ ,which can be thought of as all of the set of points, squiggles, clumps of points, and combinations thereof that can be written in a square.

Cantor demonstrated that a larger infinity can always be constructed by considering the set of all subsets of a given infinity. In general, there are 2­^M subsets of a set with M elements. For example, if a set has 3 elements, it has 2­³=2×2×2=8 subsets. These eight subsets are: A, B, C, AB, AC, BC, ABC, and the null set. Therefore, a higher infinity than ℵ_n​ is the set of all subsets of ℵ_n. ℵ_n+1 ​ is equal to 2 raised to the power of ℵ_n​​.

This means there is no largest infinity; a larger infinity can always be constructed.

Why Do We Care So Much About Infinity?

Since the time of the ancient Greeks, the enigma of infinity has been the subject of many discussions among philosophers. After the introduction of the infinity symbol in the 17th century, mathematicians began to explore the mathematical aspect of infinity through the study of infinite series, infinitely small quantities and infinitesimal calculus.

The earliest recorded idea of infinity was that of Anaximander (c. 610 - c.546 BC), a Greek philosopher. He introduced the concept of apeiron, meaning "unbounded" or "indefinite", something we know of today as "infinite". He thought of infinity as something primaeval, from which all things emerged.

Aristotle, on the other hand, did not view infinity as a completed quantity or entity. He instead differentiated potential infinity from actual infinity, arguing that sequences could be indefinitely extended without ever reaching a state of true infinity and that actual infinity did not exist in real life.

The concept of infinity evolved during the Medieval and Renaissance period. Only this time, it was viewed from a religious perspective. Thinkers like Aquinas and Duns Scotus tried to fit the concept of infinity into the framework of Christian theology. Aquinas argued that the infinite was something only the divine could embody, while Scotus believed that “if an entity is finite or infinite, it is so not by reason of something accidental to itself, but because it has its own intrinsic degree of finite or infinite perfection” (Ordinatio 1, d. 1, pars 1, q. 1–2, n. 142).

During the Enlightenment, figures like Immanuel Kant thought of infinity as something that transcended the human experience, rendering its contradictions seemingly unsolvable. He questioned whether the universe was finite or infinite, whether our world even had a beginning in time or a limit in space.

One thing is certain after examining these different viewpoints, the concept of infinity is puzzling to the human mind. Philosophy has been trying to understand it for a long time, figuring out how something could have no end and how such an idea fits into our current understanding of the world. I personally do not believe that it is even possible to visualise the thought of something continuing on forever. Though the concept of infinity is a creation of the human mind, the actual thing itself has surpassed our knowledge and comprehension. Though we may try to define it or use it to gain a perception of the vastness of the universe, I believe we will never come close to understanding what infinity is.